Rational Algebraic Expressions - amazonia.fiocruz.br

Really: Rational Algebraic Expressions

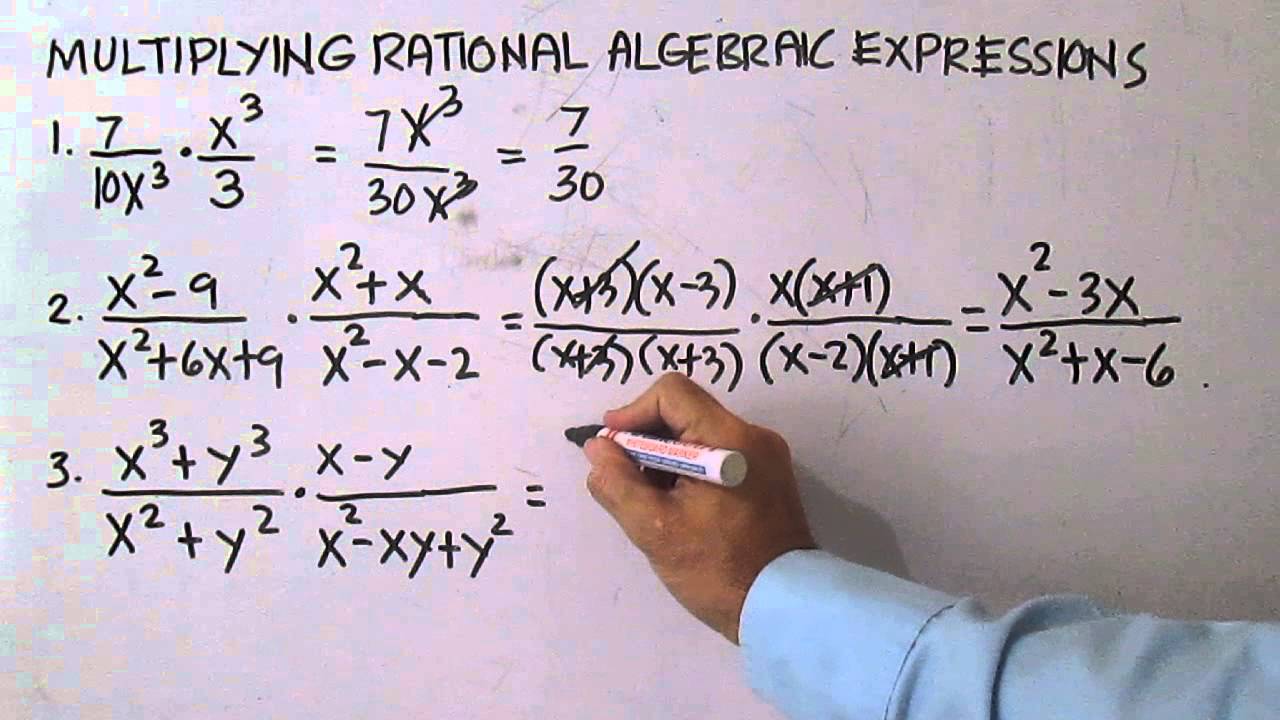
Use of Minnesota Multiphasic Personality Inventory to	Any algebraic expression that can be rewritten as a rational fraction is a rational function. While polynomial functions are defined for all values of the variables, a rational function is defined only for the values of the variables for which the denominator is not zero. 5 days ago · Multiplying And Diving Rational Algebraic Expressions - Displaying top 8 worksheets found for this concept.. Some of the worksheets for this concept are Multiplying dividing rational expressions, Multiplying rational expressions, Title multiplying and dividing rational expressions, Dividing rational expressions, Chapter 8 c multiplying and dividing an algebraic expression, Multiplying rational. 6 days ago · Aptitude test online. Use the product rule to simplify the expressions in Simplify. 28) 36 in radical form. 29) (x. In the second lesson, learner will perform operations on rational algebraic expressions, simplifiescomplex fraction, and solve problems involving rational algebraic expressions.
CATHEDRAL HILL HOSPITAL	386
Rational Algebraic Expressions	Iraq War Between Iran And Iraq
STAGES OF DEVELOPMENT A REVIEW OF THE	288
Overview Of Intellectual Property Rights	Jan 28,  · In database theory, relational algebra is a theory that uses algebraic structures with a well-founded semantics for modeling the data, and defining queries on it. The theory has been introduced by Edgar F. Codd.. The main application of relational algebra is to provide a theoretical foundation for relational databases, particularly query languages for such databases, chief among which is SQL. 2 days ago · 1. Vocabulary How can you tell if an algebraic expression is a rational expression? Simplify. Identify any x-values for which the expression is undefined. 4x6 6x2 + 13x - 5 x + 4 2. 4 days ago · Define rational algebraic expression? Any algebraic expression, that is a quotient of two other algebraic expressions, is called a rational algebraic expression. Thus if P and Q are algebraic expressions and Q ¹ 0 then the expression is called a rational algebraic expression. 3.).

Rational Algebraic Expressions

Rational Algebraic Expressions - apologise, but

Any algebraic expression, that is a quotient of two other algebraic expressions, is called a rational algebraic expression. We say that a rational algebraic expression is meaningless for those values of the variable for which the denominator Q is zero. Support the Monkey! Tell All your Friends and Teachers. Get Paid To Take Surveys!

Exponentiation is a mathematical operationwritten as b ninvolving two numbers, the base b and the exponent or power nand pronounced as " b raised to the https://amazonia.fiocruz.br/scdp/blog/culture-and-selfaeesteem/women-s-rights-by-doris-humphrey-and.php of n ". The exponent is usually shown as a superscript to the right of the base. In that case, b n is called "b raised to the Ratioanl power", "b Rational Algebraic Expressions to the power of n", [1] "the n-th power of b", "b to the n-th power", [3] or most briefly as "b to the n-th".

The definition of exponentiation can be extended to allow any real or complex exponent.

Navigation menu

Exponentiation by integer exponents can also be defined for a wide variety of algebraic structures, including matrices. Exponentiation is used extensively in many fields, including economicsbiologychemistryphysicsand computer sciencewith applications such as compound interestpopulation growthchemical reaction kineticswave behavior, and public-key cryptography. Nicolas Chuquet used a form of exponential notation in the 15th century, which was later used by Henricus Grammateus and Michael Stifel in the 16th century.

The word exponent was coined in by Michael Stifel. Some mathematicians such as Isaac Newton used exponents only for powers Rational Algebraic Expressions than two, preferring to represent squares as repeated multiplication.

Another historical synonym, involutionis now rare [15] and should not be confused with its more common meaning. It is clear that quantities of this kind are not algebraic functionssince in those the exponents must be constant.

When it is a positive integerthe exponent indicates how many copies of the base are multiplied together. The base 3 appears 5 times in the repeated multiplication, because the exponent is 5. Here, is the 5th power of 3or 3 raised to the 5th power. The word "raised" is usually omitted, and sometimes "power" as well, so 3 5 can be simply read "3 to the 5th", or "3 to Algebraif 5".

Therefore, the exponentiation b n can be Rational Algebraic Expressions as " b to the power of n ", " b to the n th power", " b to the n th", or most briefly as " b to the n ". The exponentiation operation with integer exponents may be defined directly from elementary arithmetic operations. Powers with positive integer exponents may be defined by the base case [17].

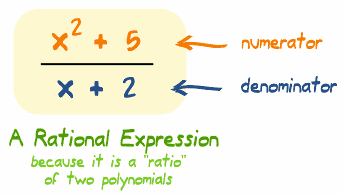
The associativity of multiplication implies that for any positive integers m and n. Any nonzero number raised to the 0 power is 1 : [18] [2]. One interpretation of such a power is as an empty product. The case of Ratoonal 0 is more complicated, and the choice of whether to assign it a continue reading and what value to assign may depend on context. For more details, see Zero to the power of zero.

The following identity holds for any integer n and nonzero b :. The identity above may be derived through a definition aimed at extending the Rational Algebraic Expressions of exponents to negative integers.]